(멀티플 뷰 지오메트리) Lecture 11. Two-view and multi-view stereo
2022, Apr 20
- 참조 : https://youtu.be/OpZs7kfjFPA?list=PLxg0CGqViygP47ERvqHw_v7FVnUovJeaz
- 참조 : https://youtu.be/N_iECTqZtQQ?list=PLxg0CGqViygP47ERvqHw_v7FVnUovJeaz
- 참조 : https://youtu.be/hfQR7X8eH9s?list=PLxg0CGqViygP47ERvqHw_v7FVnUovJeaz
- 참조 : https://youtu.be/YgOC9DjHyJs?list=PLxg0CGqViygP47ERvqHw_v7FVnUovJeaz
- 참조 : Multiple View Geometry in Computer Vision




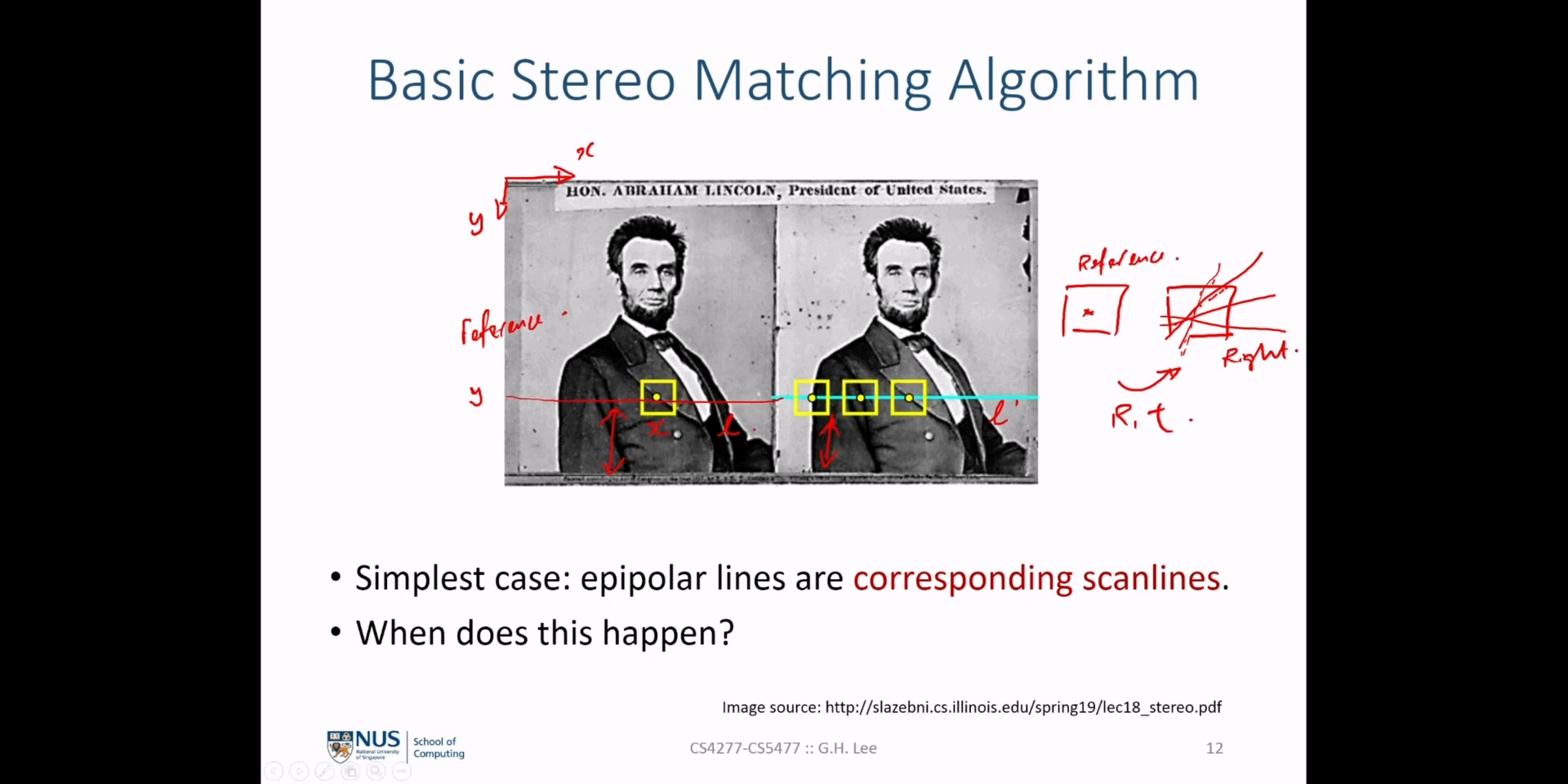
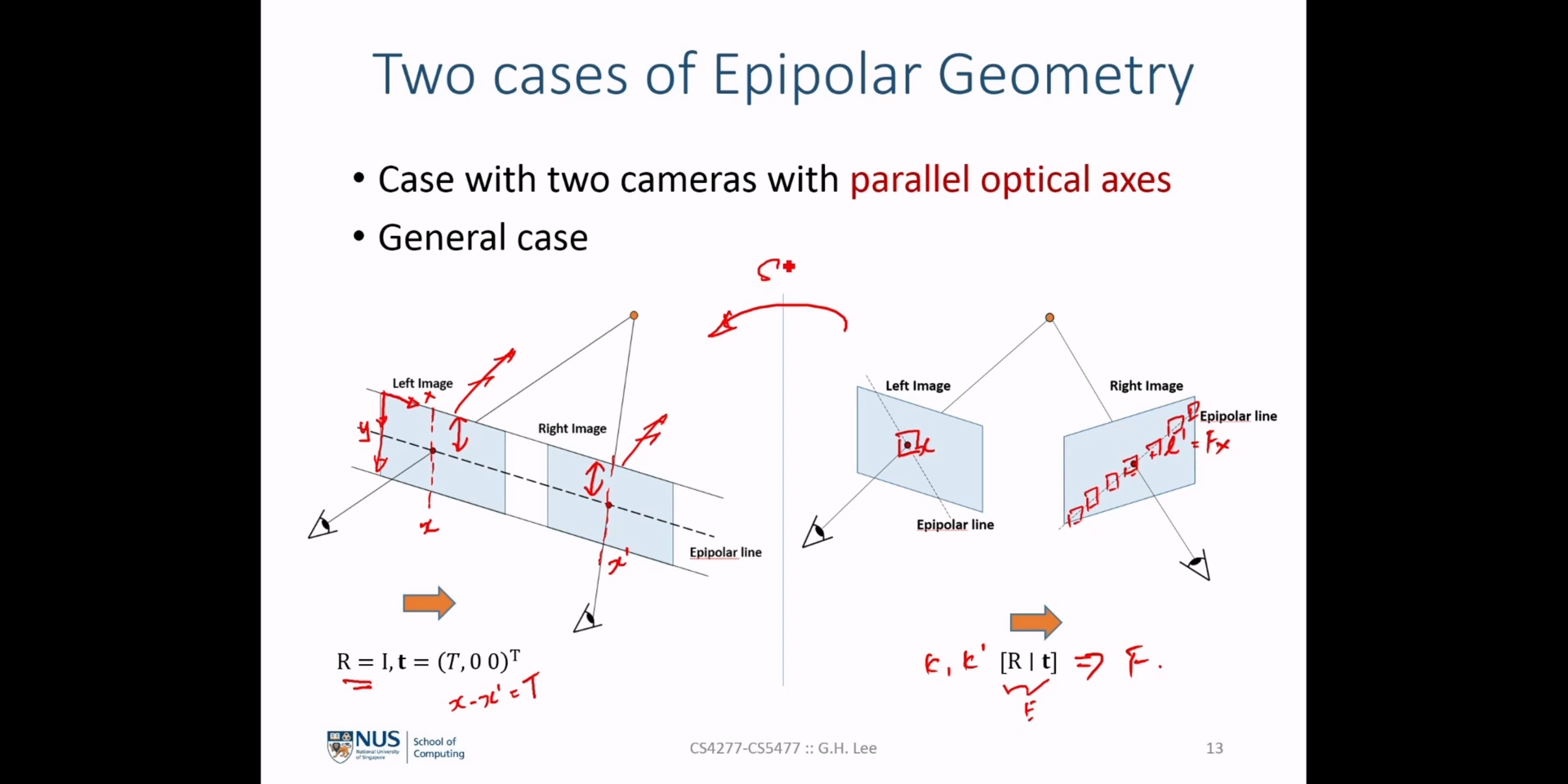
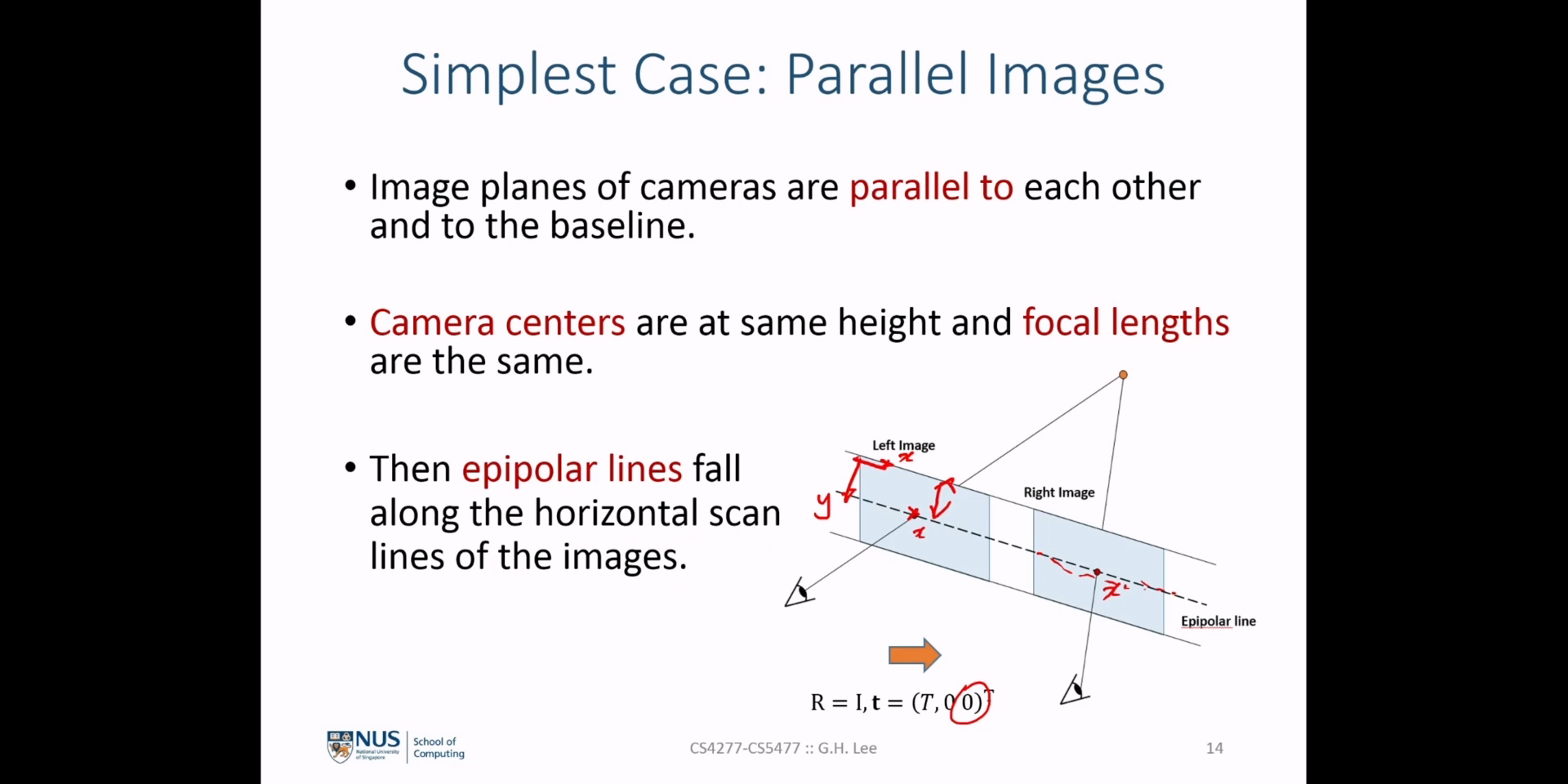
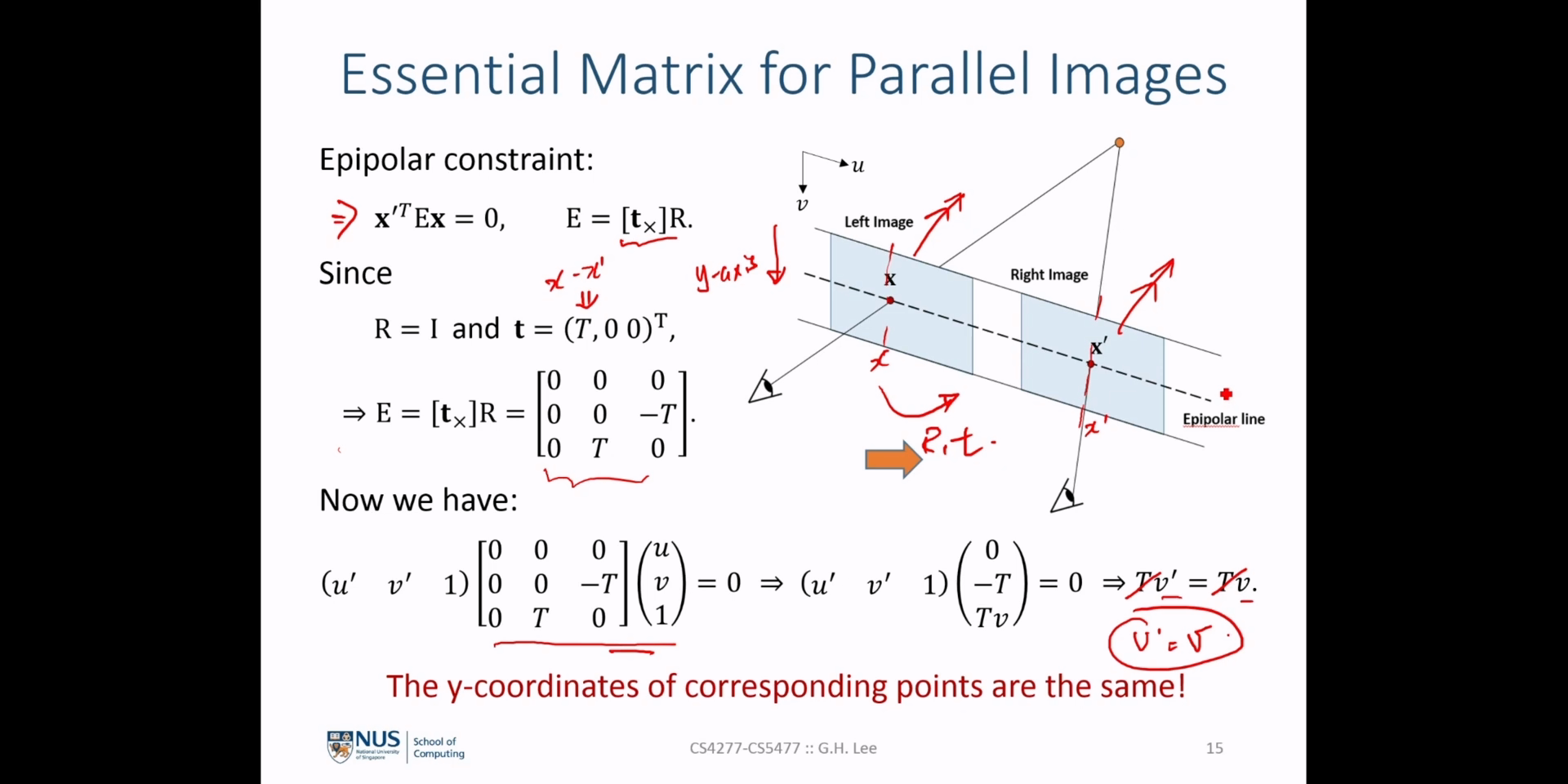
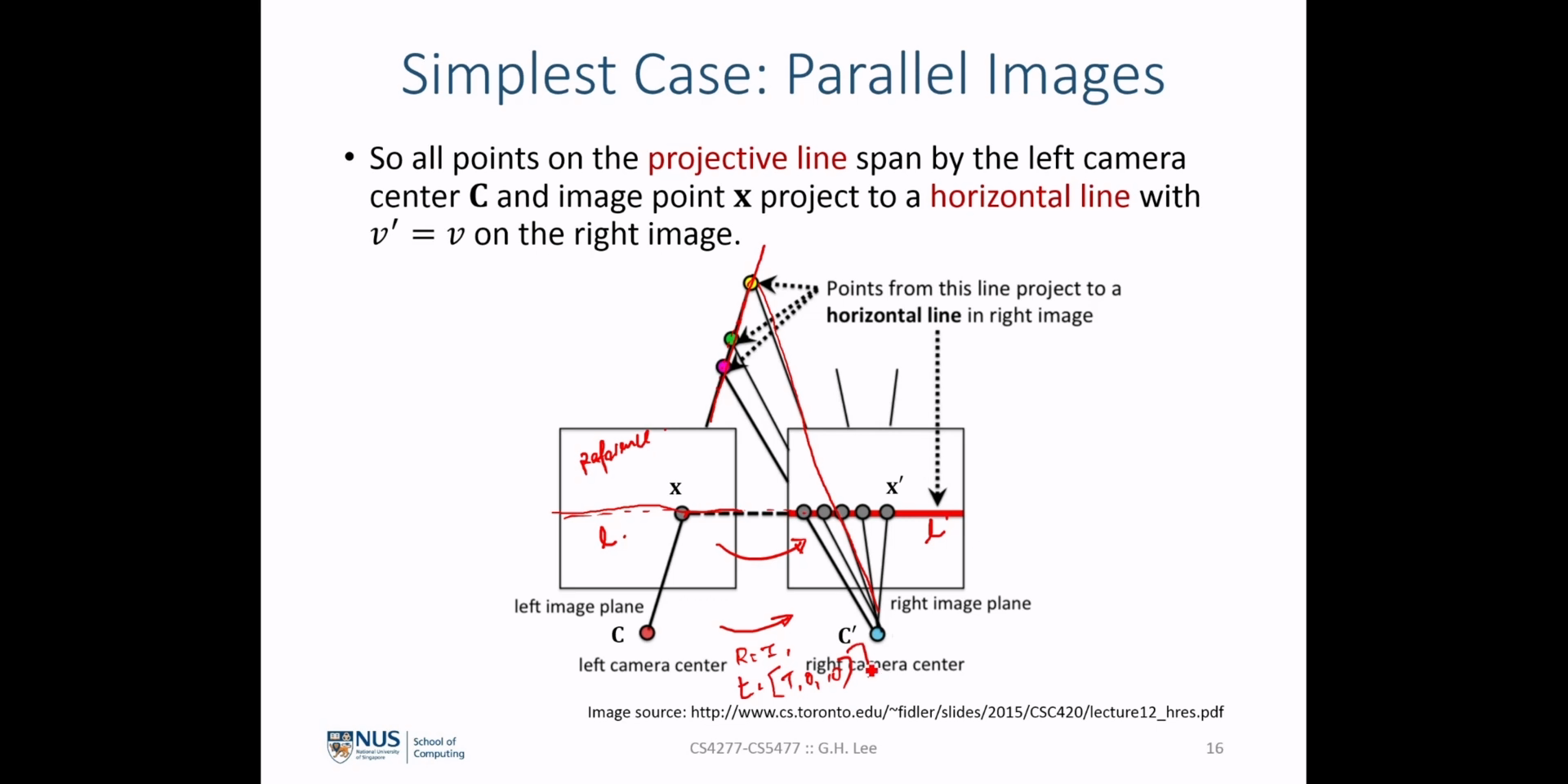
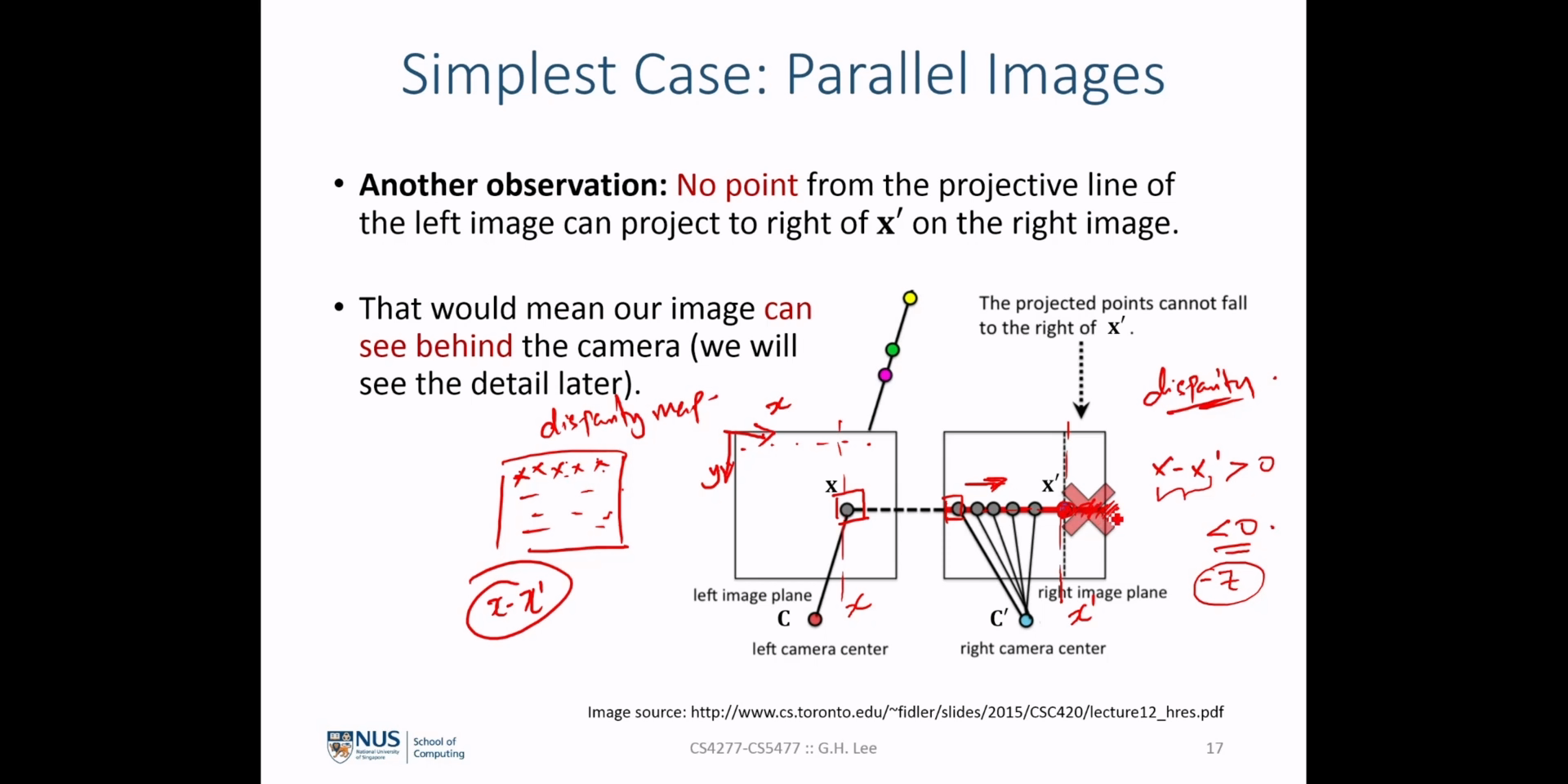
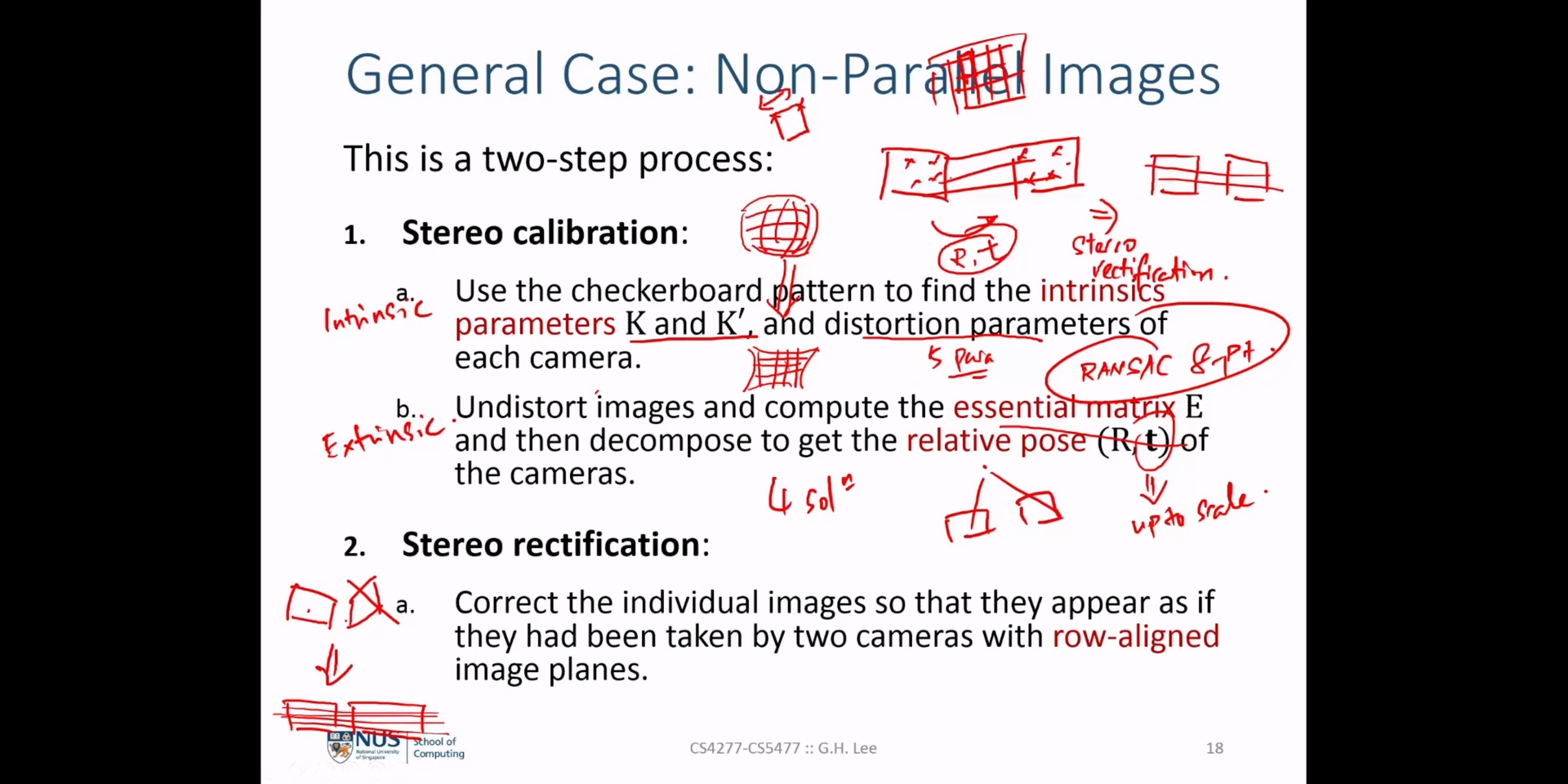
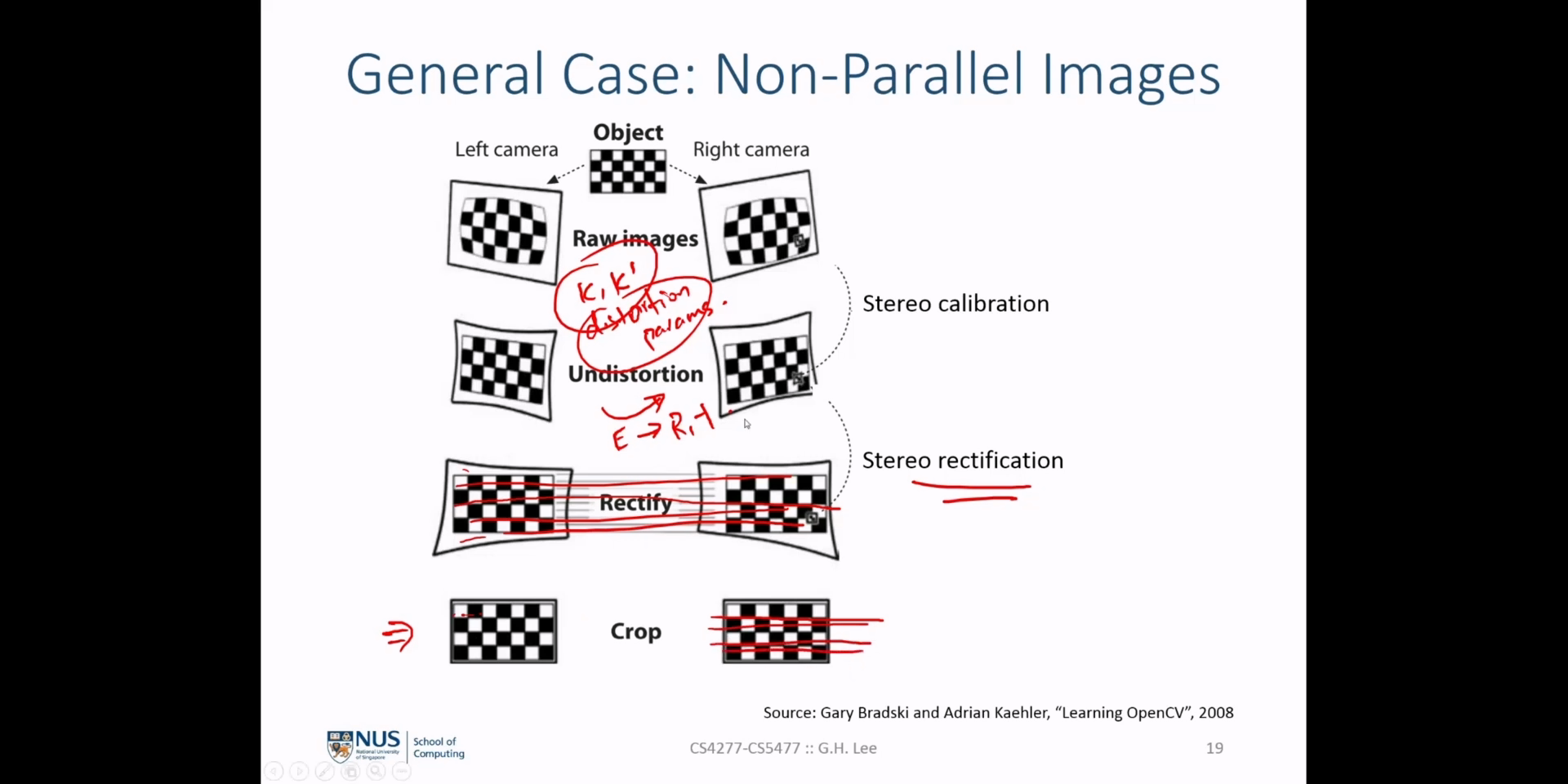
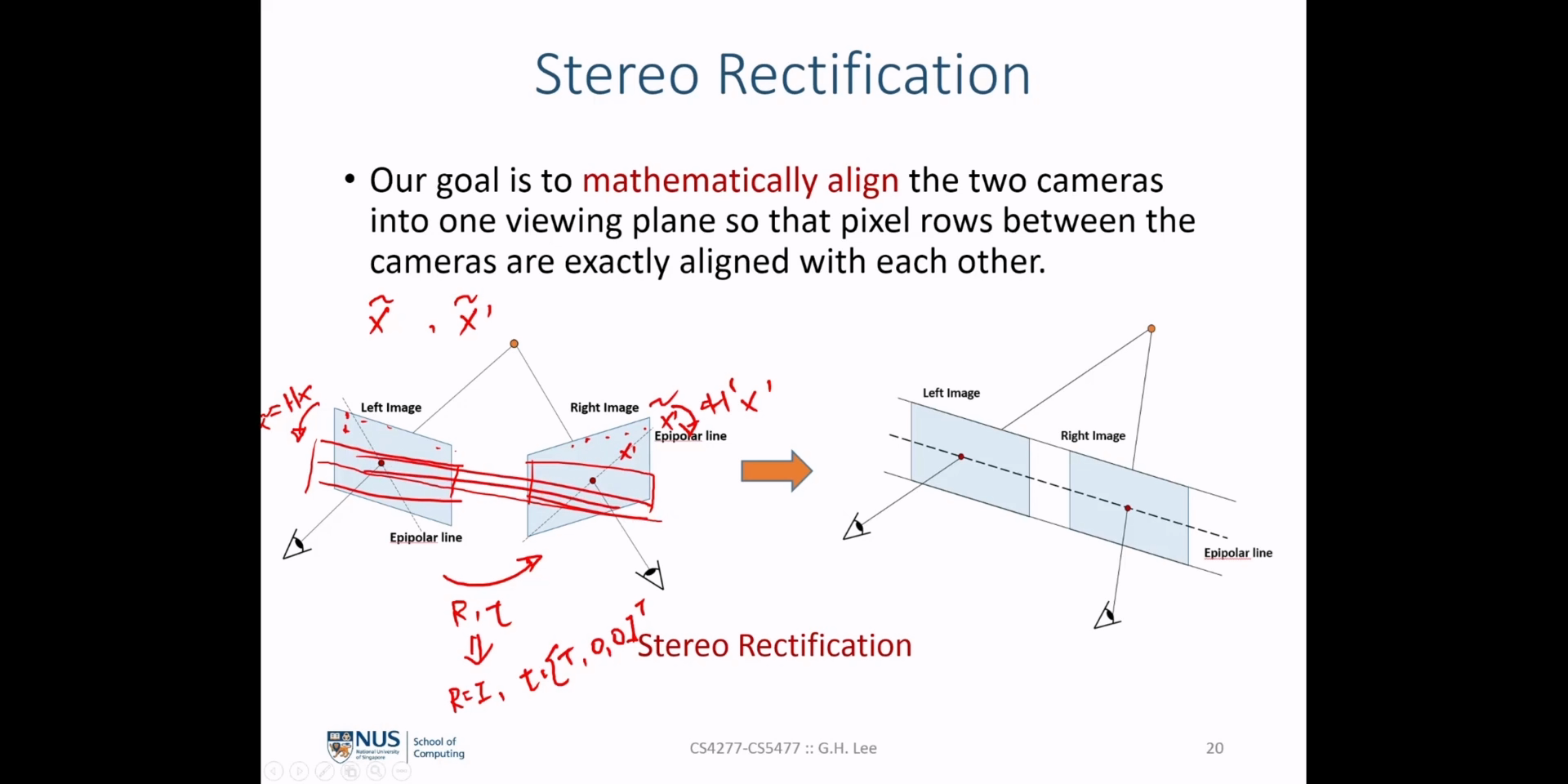
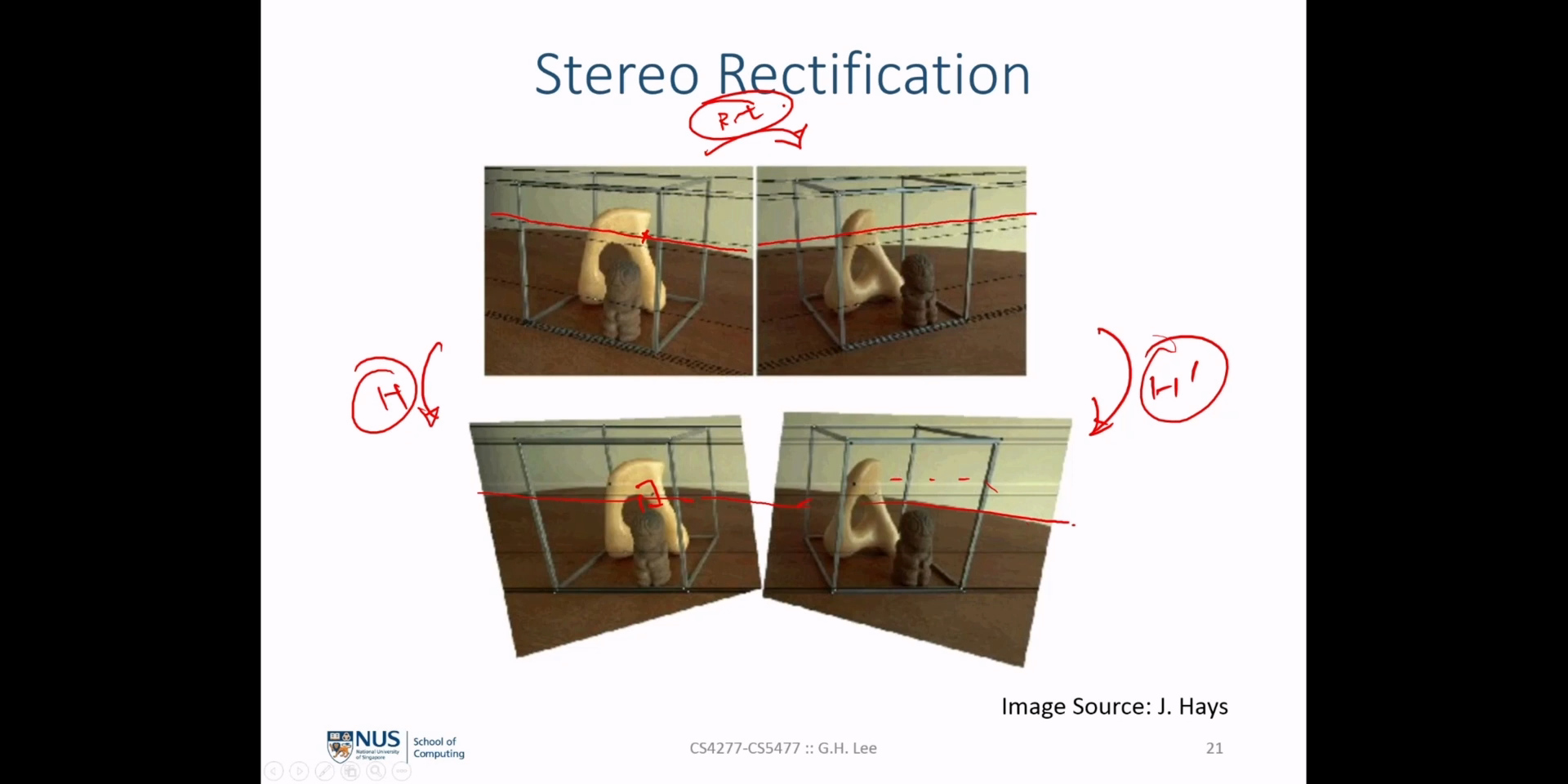
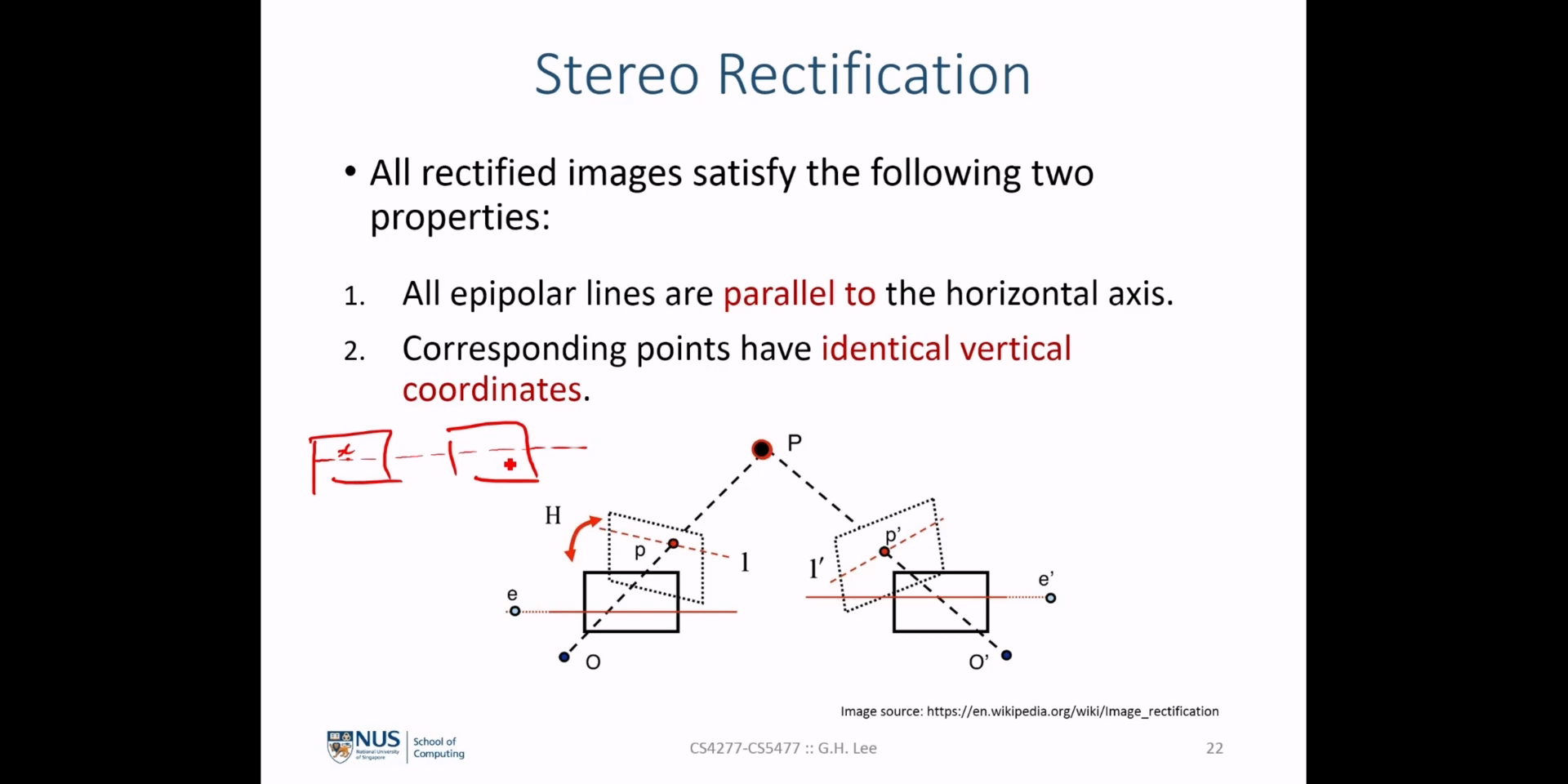
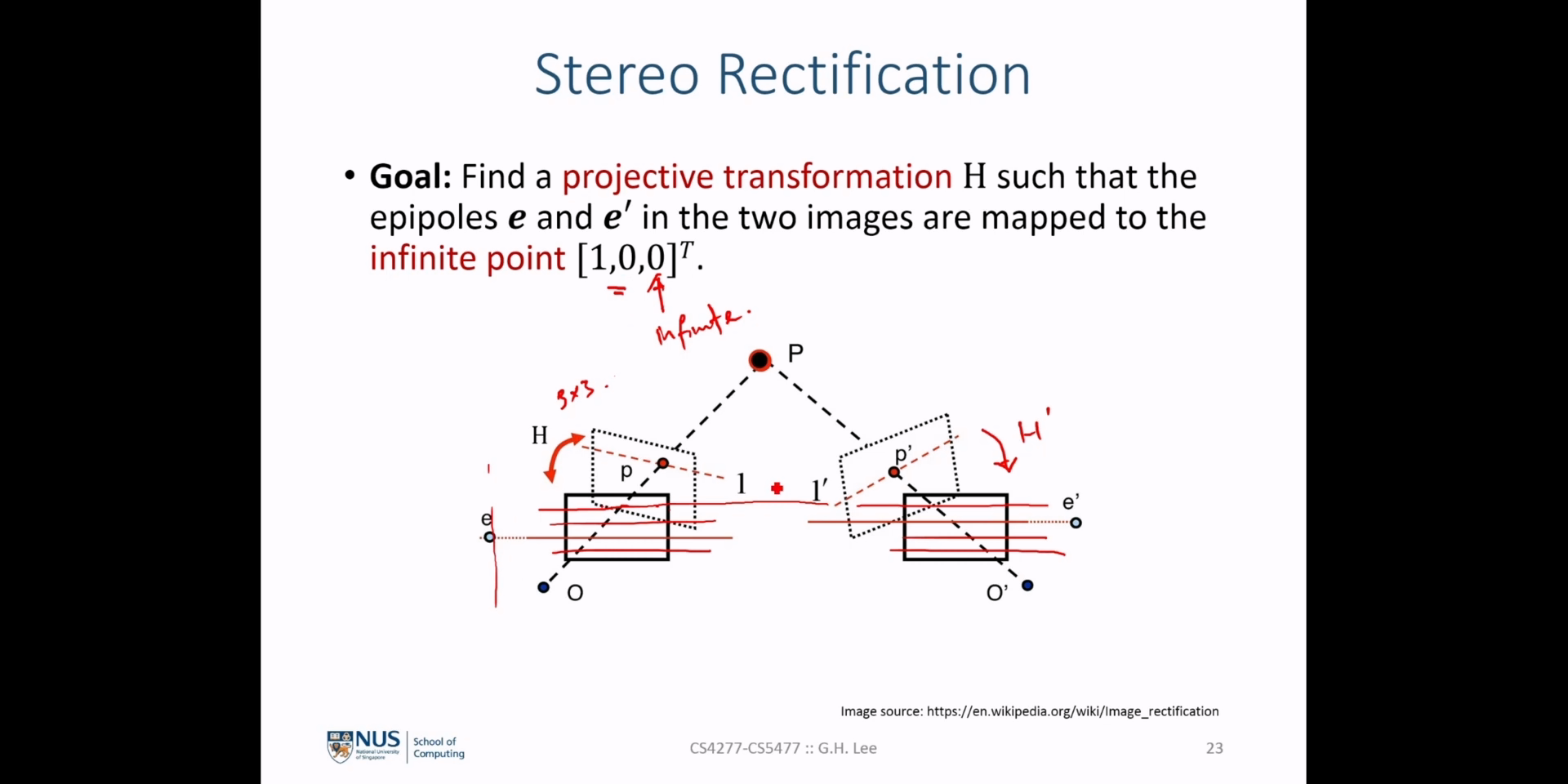
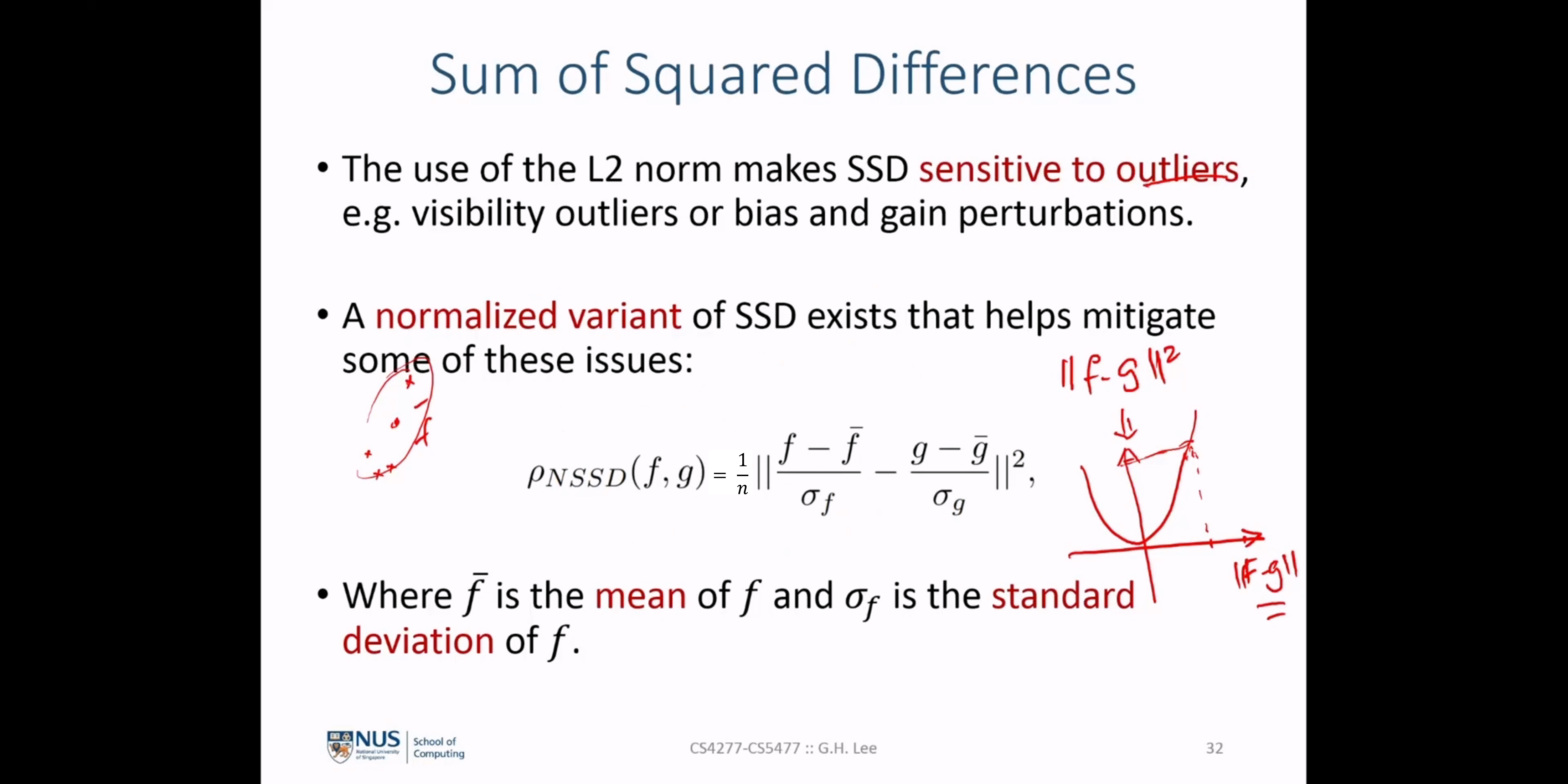
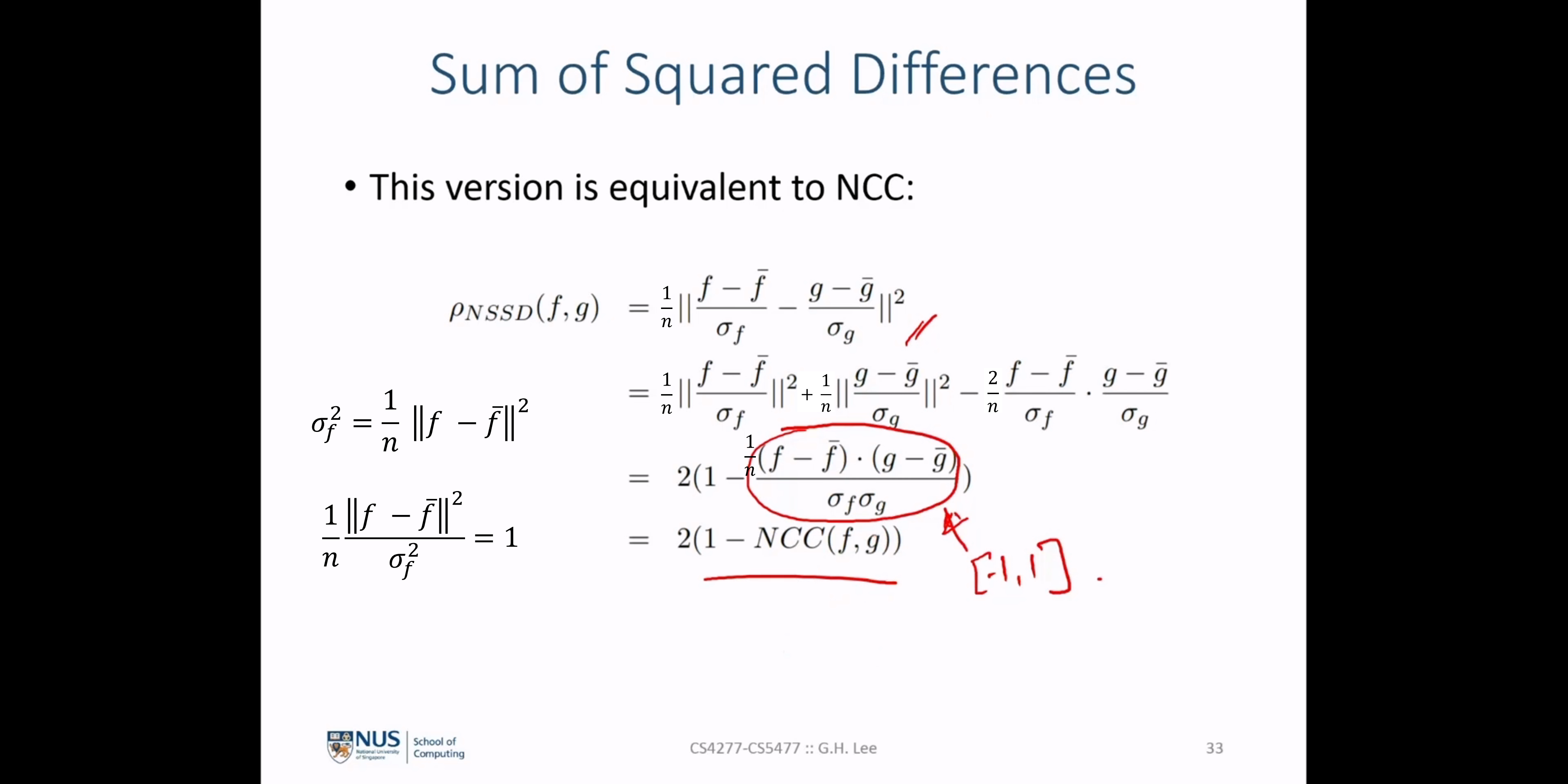
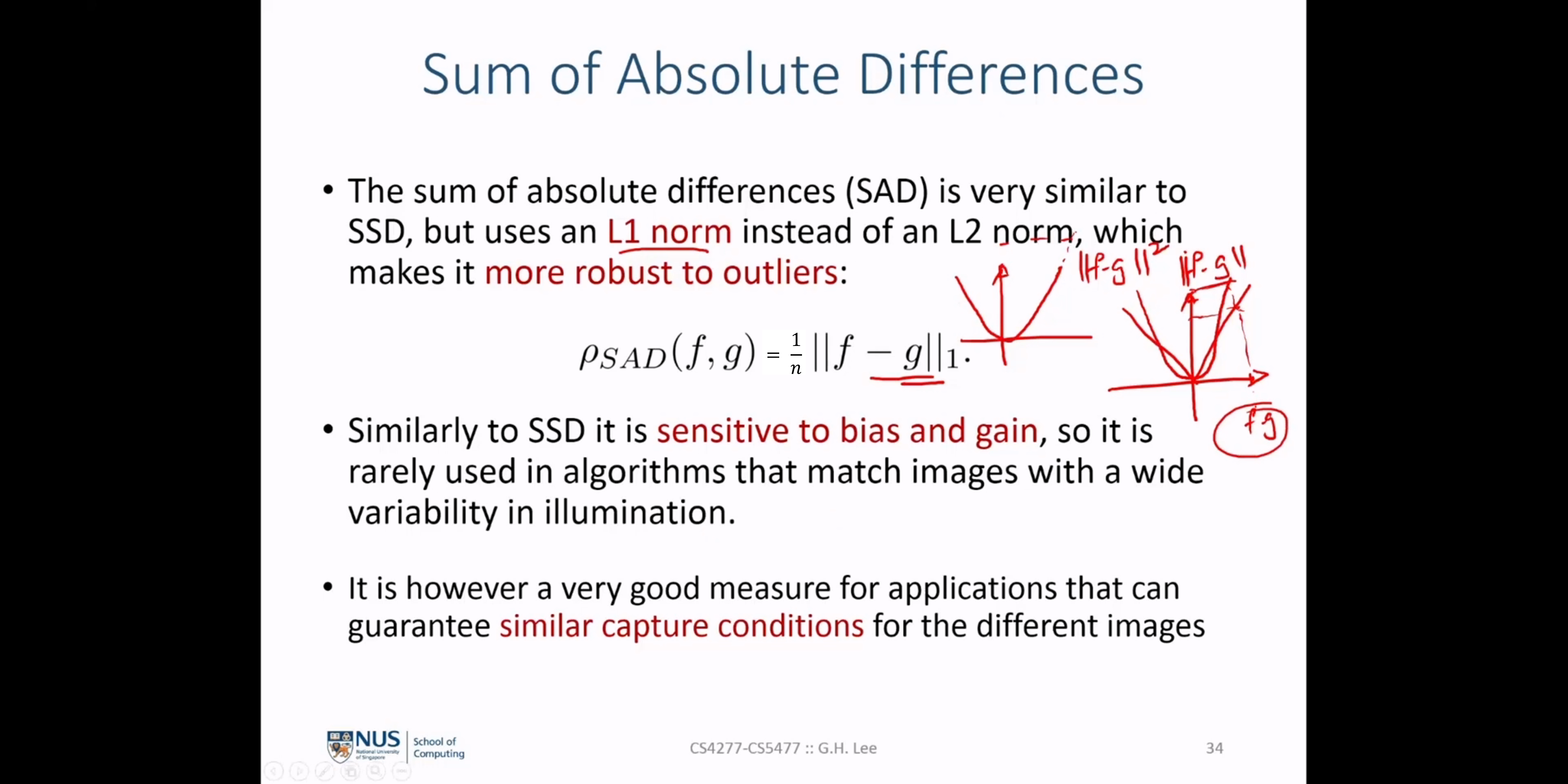
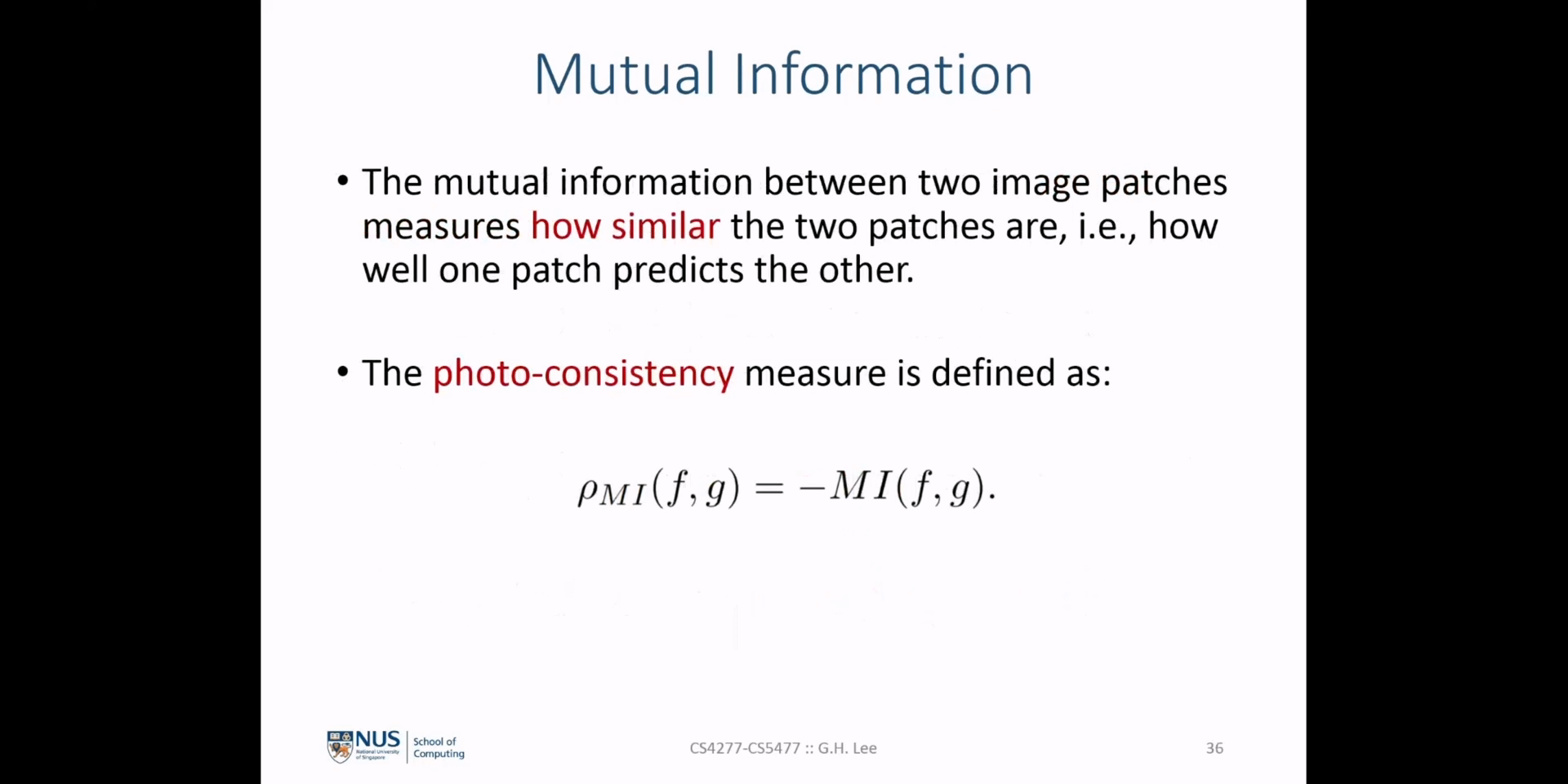

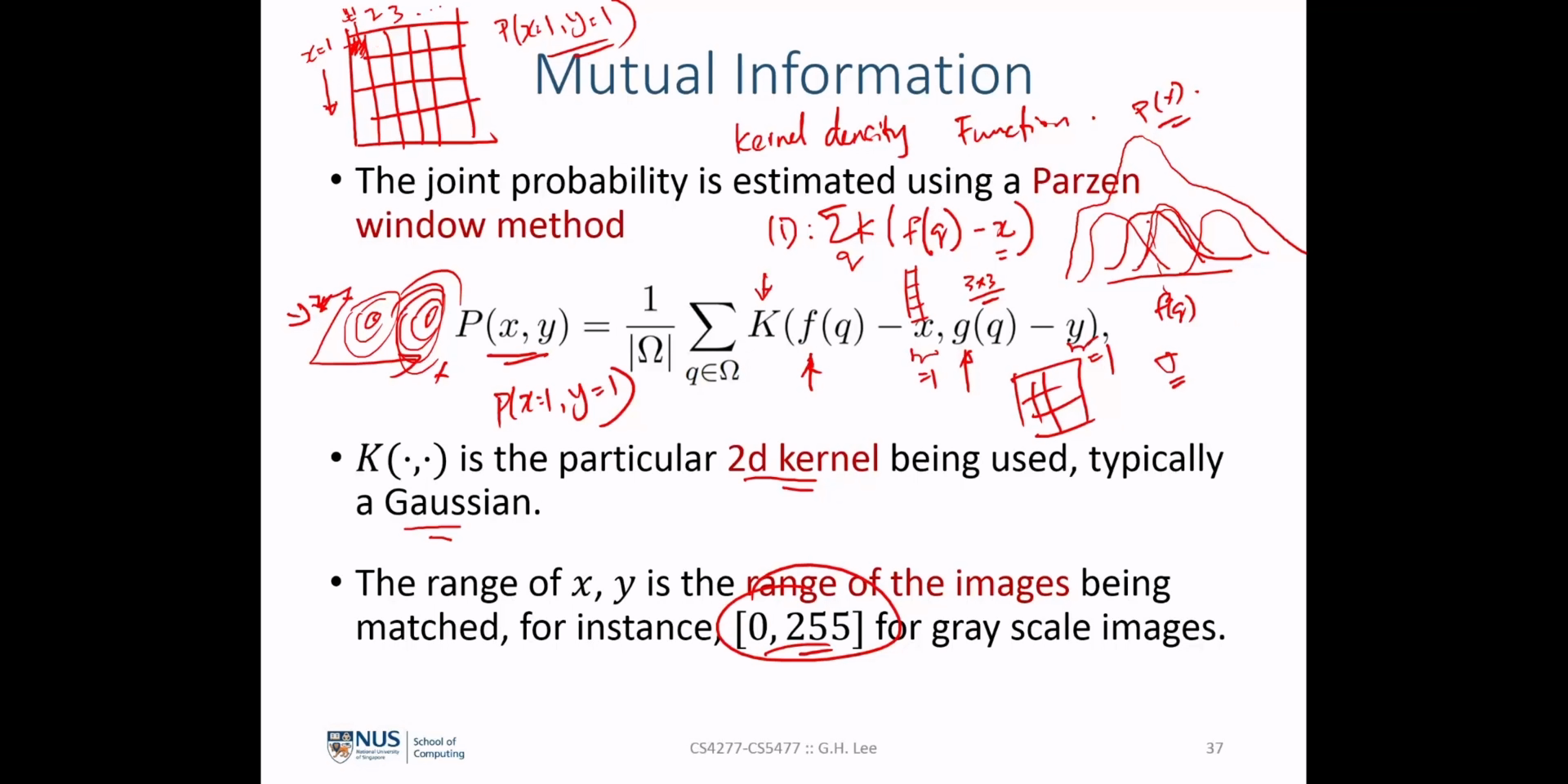
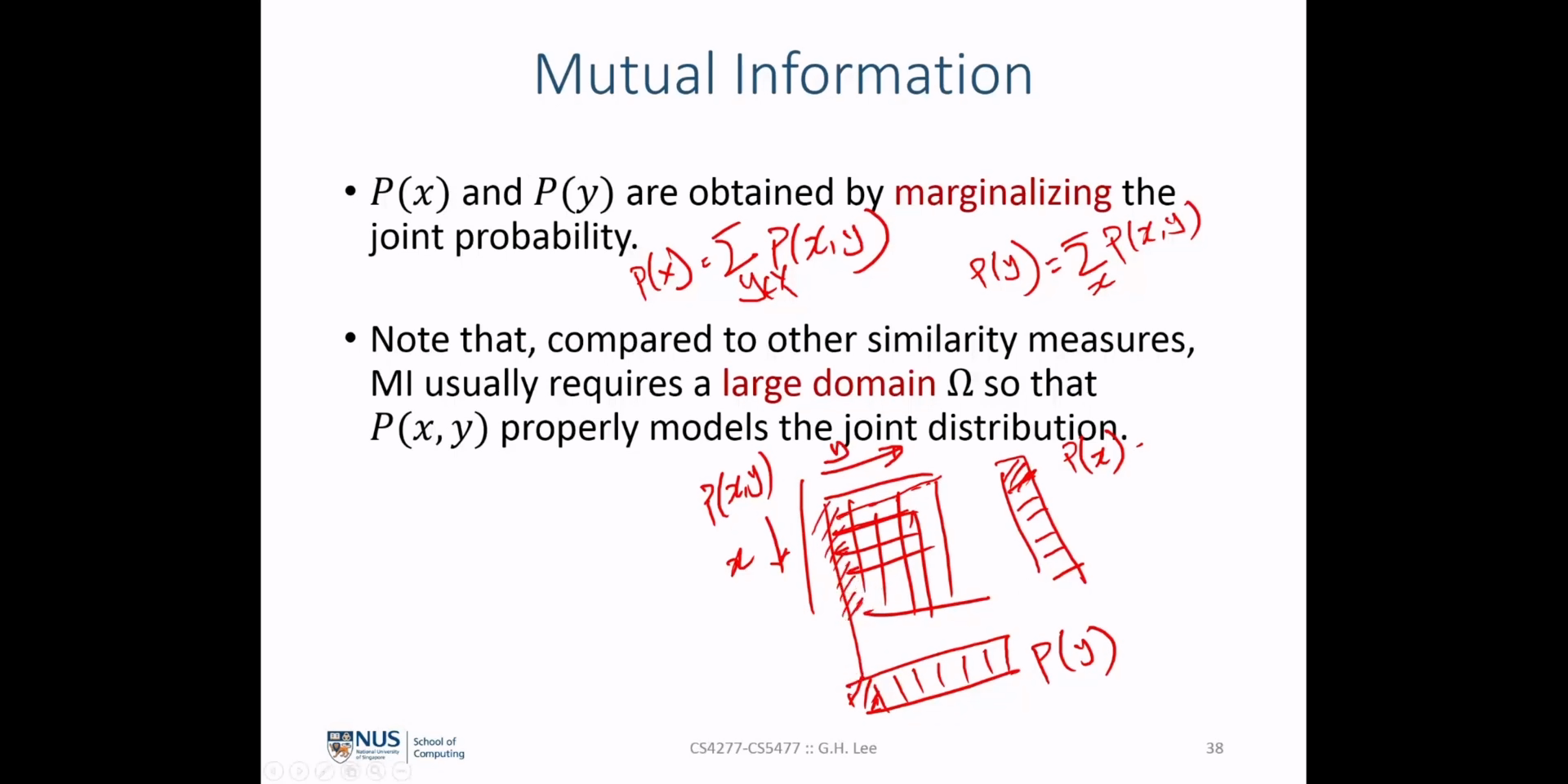
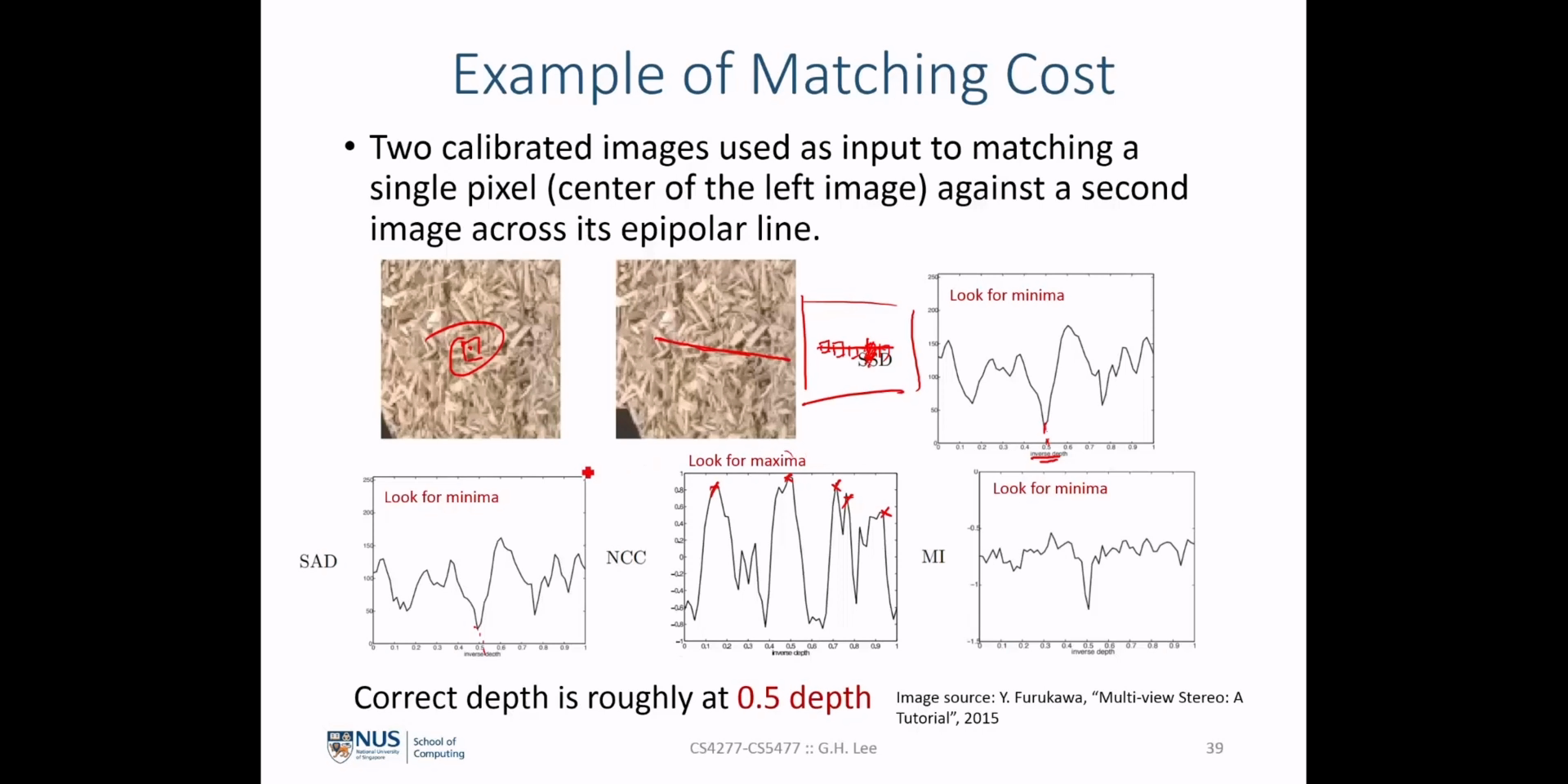


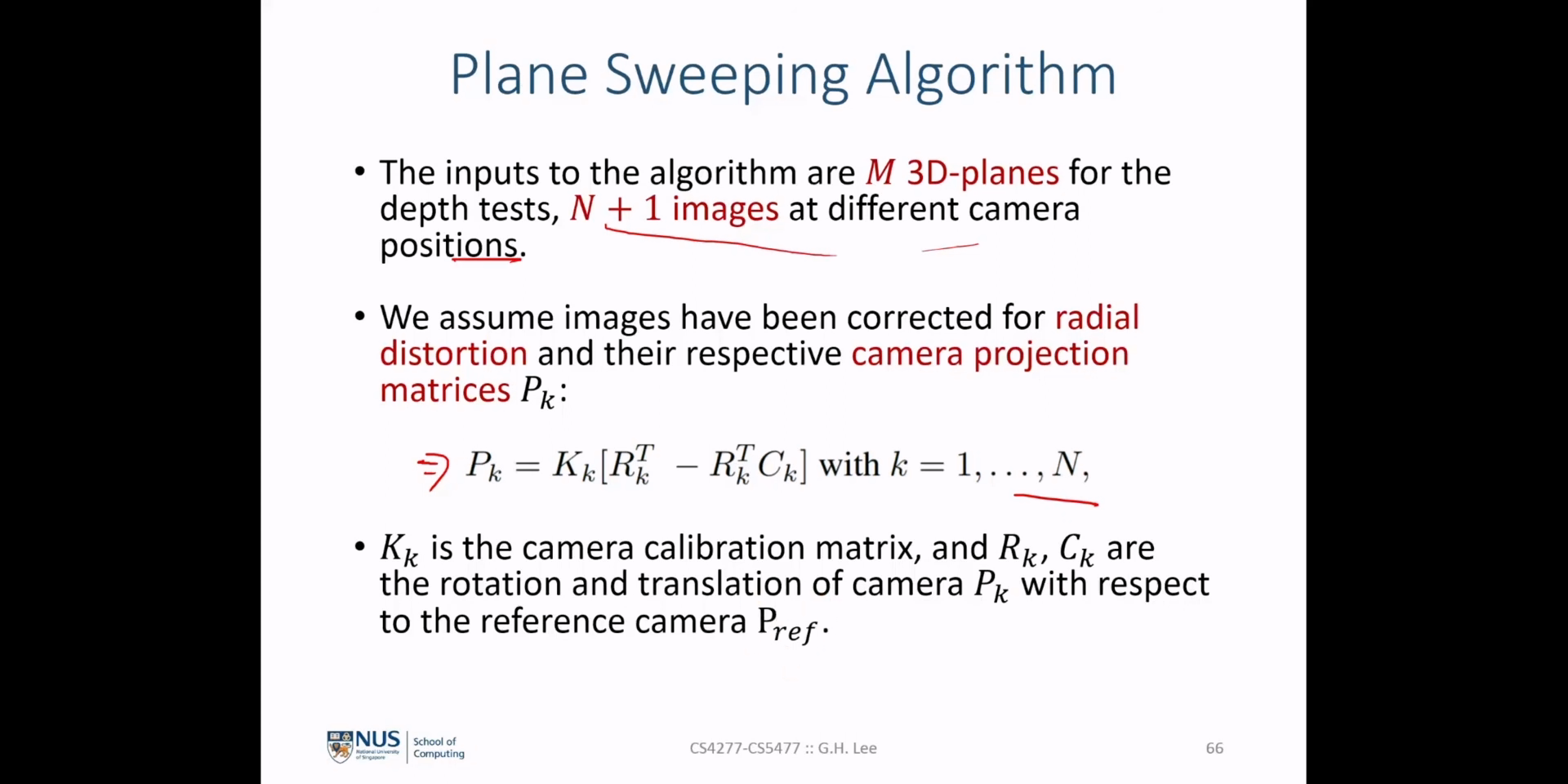
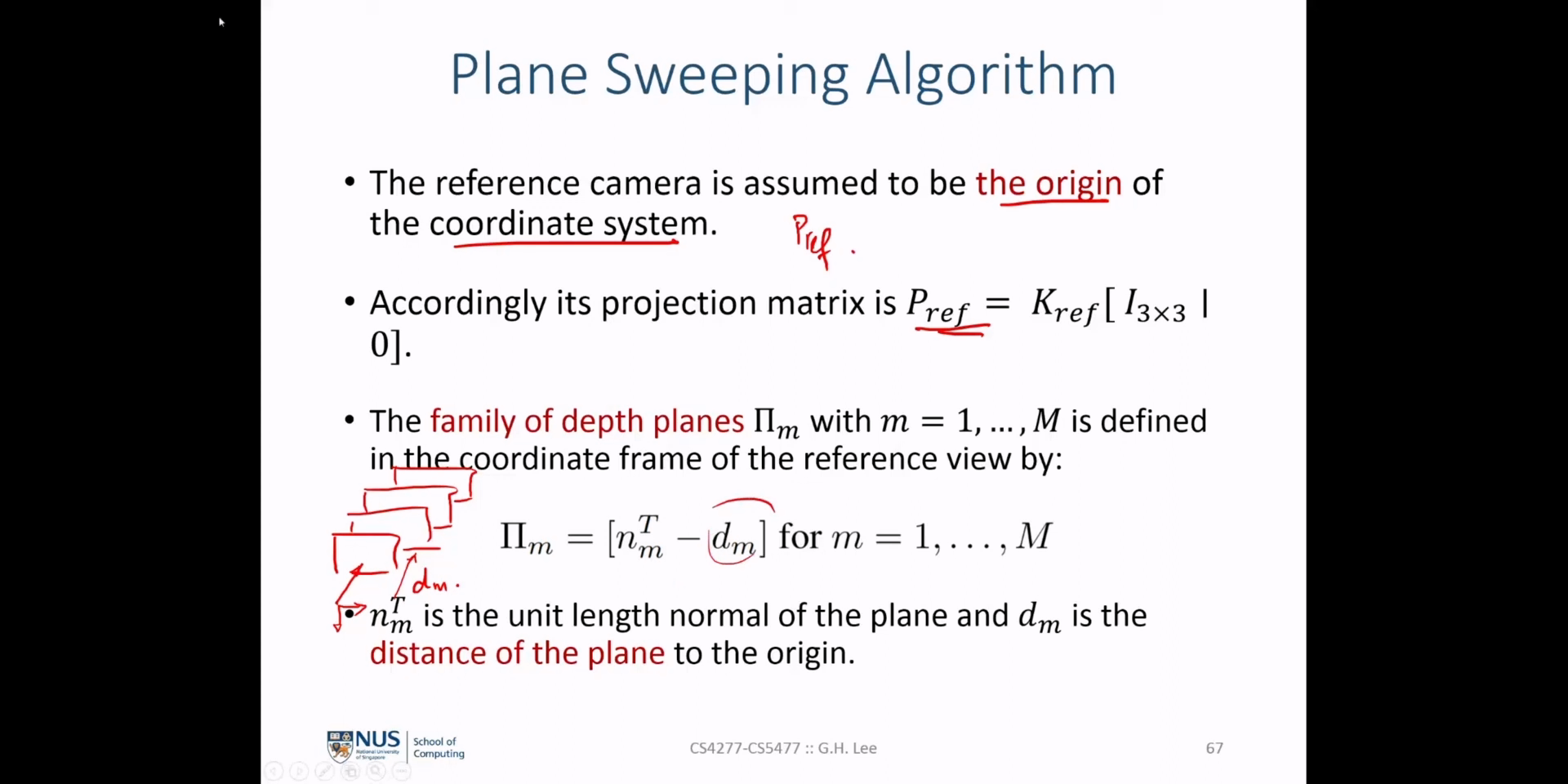
- Reference Camera Projection Matrix: Pref=Ktextref[I|0]Reference Camera Projection Matrix: Pref=Ktextref[I|0]
- Camera k Projection Matrix: Pk=Kk[RTk|−RTkCk]Camera k Projection Matrix: Pk=Kk[RTk|−RTkCk]
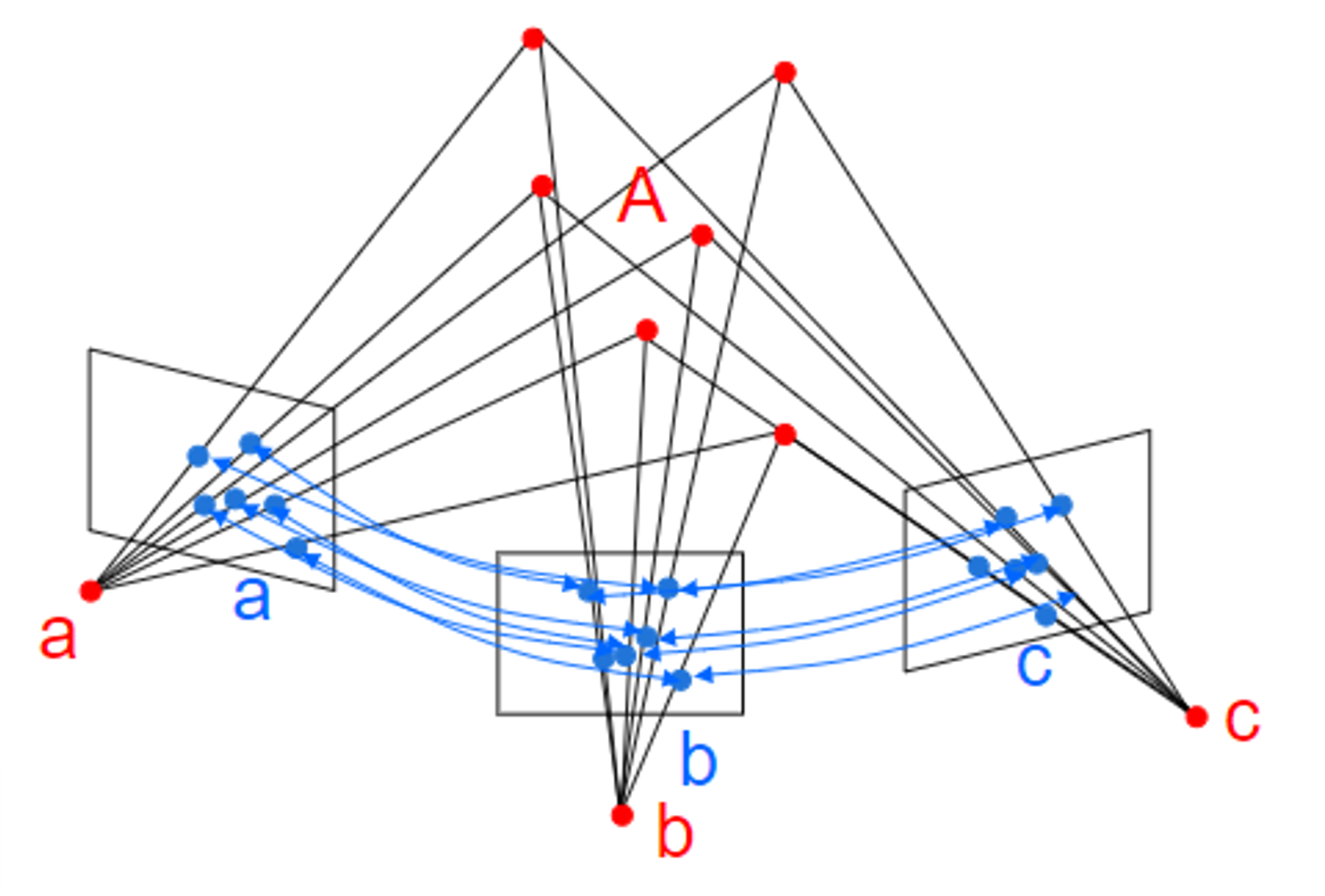
reference camera의image plane으로 부터 카메라 kk 의image plane으로 매핑하는homography를 구해보도록 하겠습니다.reference camera image plane상의 임의의 점을 xrefxref 라고 하고 이에 대응되는 kk 카메라의image plane상의 점을 xkxk 라고 하겠습니다.
- X: A point on the Πm plane in homogeneous coordinates.X: A point on the Πm plane in homogeneous coordinates.
- nTmX−dm=0nTmX−dm=0
- Point XX 를 xrefxref 와 xkxk 로 투영하면 다음과 같습니다.
- Reference Camera: xref=PrefX=KrefXReference Camera: xref=PrefX=KrefX
- Camera K: xk=PkX=Kk(RTkX−RTkCk)Camera K: xk=PkX=Kk(RTkX−RTkCk)
- 위 xref,xkxref,xk 식을 변형하면 다음과 같습니다.
- X=K−1refxrefX=K−1refxref
- nTmX=dmnTmX=dm
- nTmK−1refxref=dmnTmK−1refxref=dm
- nTmK−1refxrefdm=1 : This term will be used below.nTmK−1refxrefdm=1 : This term will be used below.
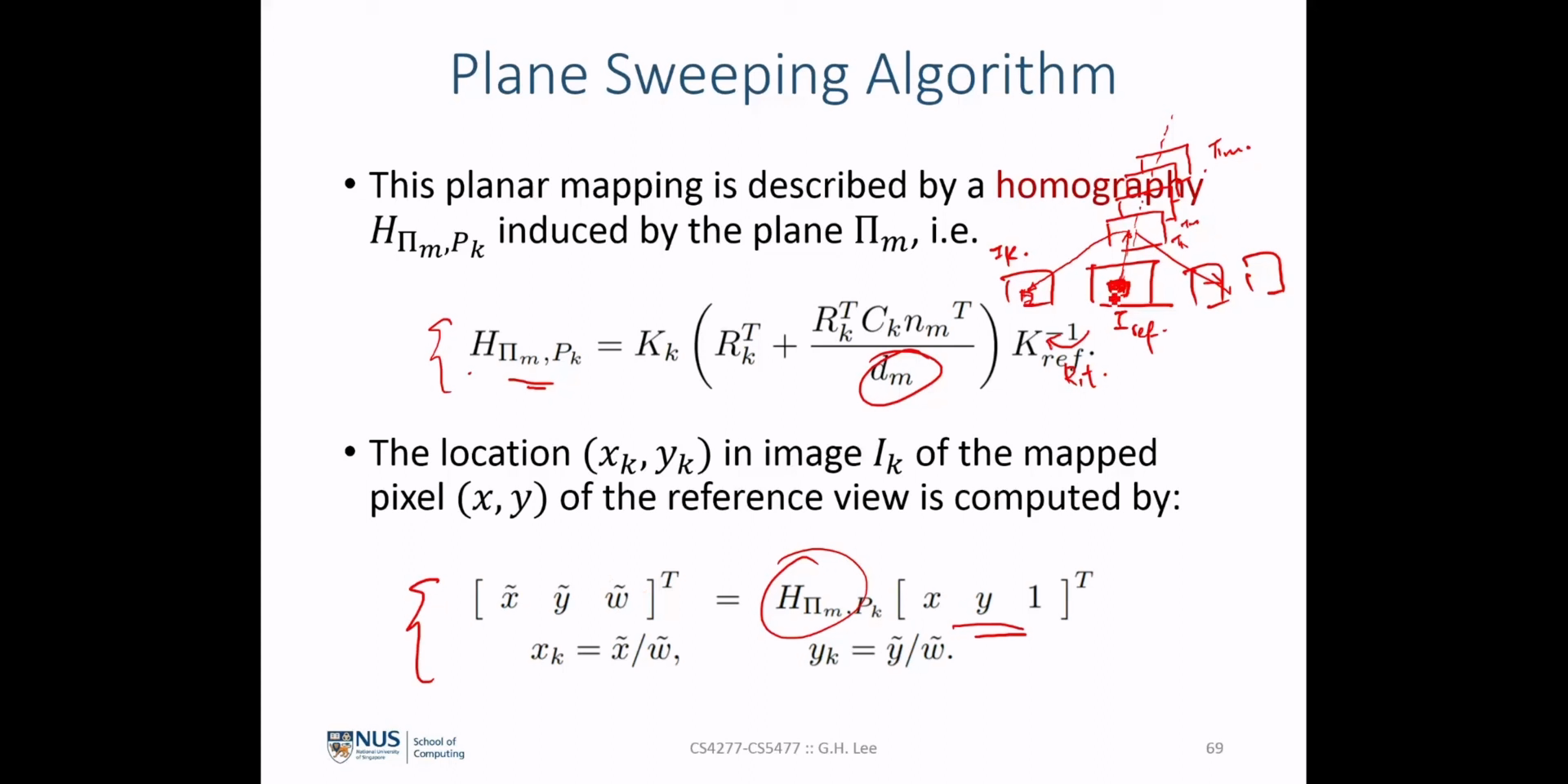
- xk=Kk(RTkK−1refxref−RTkCk)=KkRTkK−1refxref−KkRTkCk=KkRTkK−1refxref−KkRTkCk⋅nTmK−1refxrefdm(∵nTmK−1refxrefdm=1)=Kk(RTk+RTkCknmdm)K−1refxref
- 즉, xref 를 xk 로 변환하는
Homography인 HΠm,Pk 는 위 파란색 식으로 정의될 수 있습니다.
- HΠm,Pk=Kk(RTk+RTkCknmdm)K−1ref